Background
Propellant management devices, or PMDs, are often used within the fuel and oxidizer tanks of flight vehicles to prevent or delay vapor ingestion into the propulsion system. In space, when the location of gas and liquid within a tank is no longer dictated by gravitational forces, there is a risk of ingesting the gaseous phase into the propulsion system and rendering the system inoperable. PMDs are effective because they rely on surface tension to ensure that the liquid propellant is always available to the propulsion system.
The design of PMDs relies almost exclusively on analysis with experimental testing being limited or unfeasible. If viable, a common experimental form of testing is drop tower testing which takes a scaled model of the tank and drops it from great heights to produce just a few seconds of micro-gravity behavior. Because of the brief window and scaling required, drop tower testing is commonly used only to confirm expected static configurations; however, there are risks in the design of PMD’s that warrant looking at dynamic performance. This project utilizes the concept of neutral buoyancy, which is the balancing of gravity and buoyancy forces using equally dense immiscible fluids, to reproduce transient micro-gravity effects on fluid behavior in a ground-based laboratory. The appeal of using a neutral buoyancy approach is that it requires no scaling, can hold a micro-g configuration indefinitely, and can be tested for dynamic situations.
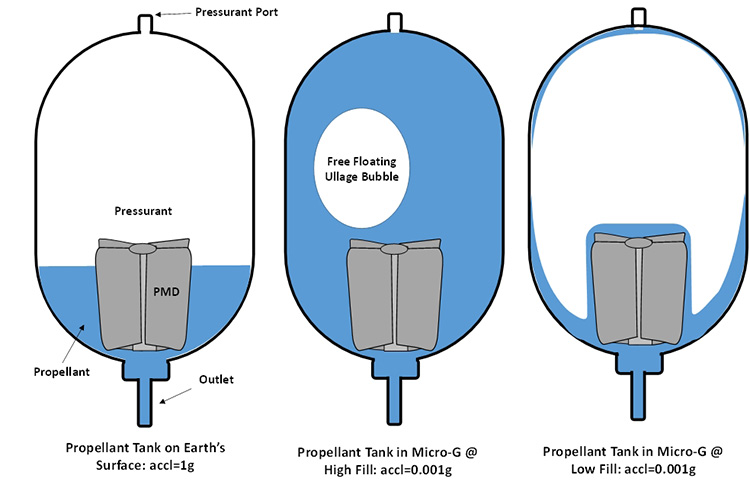
Figure 1: Propellant tank example and scenarios.
Approach
A plan was devised to create a novel neutral buoyancy PMD test bench. The first task was to select safe, immiscible fluids that can accurately replicate the fluid properties of liquid oxygen in the neutral buoyancy context. Liquid oxygen is a high-performance oxidizer that is critical to future exploration architectures but there is no documented experience with liquid oxygen PMDs, making it of particular interest. The second task was to design a representative tank and PMD to be used as the first test case for the new neutral buoyancy test bench (NBT). The final task was to construct the neutral buoyancy test stand and simulate dynamic events at the PMD, such as tank draining under non-axial micro-gravity accelerations. As an intermediary step between testing a full-scale tank and PMD, a subscale component-level dynamic test was performed looking at steady state flow through a wedge. Experimental data exists for validation, and additional steady-state vane flow was analyzed within standard micro-gravity fluid analysis software, Surface Evolver (SE). This modeling accomplishment is novel itself, provided that Surface Evolver is an exclusively static-based solver and new methods will be applied to extend it to non-static conditions.

Figure 2: Surface evolver simulations for test apparatuses. (left) Steady State flow through a 5-degree wedge. (right) Static surface evolver simulation at a lateral 100 micro-g and 2% fill.
Accomplishments
The project has yielded a solvent mixture capable of simulating liquid oxygen (LOX) fluid properties and achieving density matching to a level that will produce simulated accelerations of up to 40 micro-g. Neutral buoyancy tests performed in a full-scale tank provided insight on overall PMD performance and complex fluid interactions. One such comparison was made for a simulated lateral acceleration on a tank with 10% fill in a 185 µg environment. Figure 3 shows a strong similarity of the fluid surface between what was observed in the NBT and what the Surface Evolver model predicts for the same tank under the same conditions.

Figure 3: Lateral acceleration at a 10% tank fill and simulated acceleration of 185 µg. Left: NBT, Center: Surface Evolver from experiment POV, Right: Surface Evolver cross-section view.
A typical study performed when designing PMD’s at SwRI is to study the interaction between the volume of propellant within the sponge to that of the liquid pool and vane. In one such instance, the vane and sponge liquid bridge can be observed breaking during a static non-flowing case as shown in Figure 4.
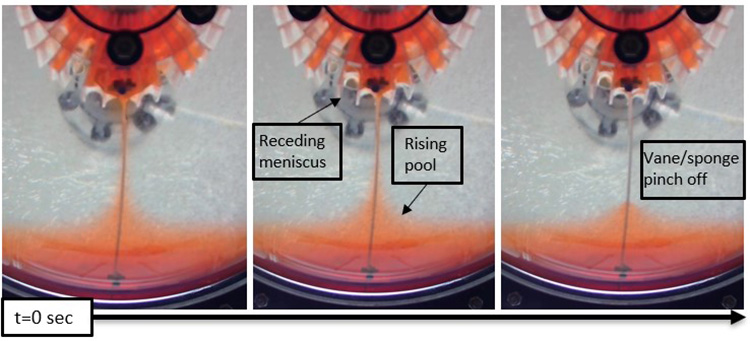
Figure 4: Vane and sponge detachment under static lateral acceleration during a non-flowing case. Left to right shows the progressive fluid transfer from an unstable state to a stable one.
Lastly, the component level wedge test showed strong agreement for a steady state flow below a certain rate. Figure 5 shows the 5° wedge test at a 32% fill under varying low flow rates. The NBT surface (orange) is overlayed by the SE surface (black). Strong agreement can be observed until a flow rate of around 180 mL/min is reached, where the inlet/outlet effects become more apparent and diverge from the model’s conditions.

Figure 5: Surface Evolver overlay of the 5° wedge test at 32% fill under varying low flow rates.
